Heavy-ion collisions (AA) at ultrarelativistic energies at the RHIC and LHC have produced during the last 25 and 15 years, respectively, an extremely hot and dense environment, a strongly interacting coloured medium named “quark-gluon plasma” (QGP), a state of matter where quarks and gluons are not confined anymore in neutral-coloured hadrons [1,2]. The LHC experiments, and particularly ALICE, are interested in the measurement of the QGP properties, including its volume, density, temperature, and lifetime. Taken together, these measurements help define the QGP’s equation of state.

To accomplish these measurements, heavy-ion physicists rely on different kinds of probes, in particular, “hard probes”: high-energy particles (roughly above 5 GeV) that include quarks, gluons, and electroweak bosons such as Z⁰, W^±, and prompt photons. Produced in the earliest moments of the collision—before the QGP forms—these energetic particles pass through the hot nuclear matter like bullets, providing valuable insights into the QGP’s properties.

Partons interact strongly with the medium, while electroweak bosons do not, escaping the QGP unmodified. Partons will “see” the coloured medium losing energy while traversing it via radiational (gluon radiation) and collisional processes — an effect known as “jet-quenching” [3,4]. Measuring this parton energy loss is one of the main aims of heavy-ion experiments since it provides insights into, for example, the path length partons travel through the medium and its energy density. If electroweak bosons do not interact strongly, why are they interesting for heavy-ion physics?

Prompt photons to study the QGP as a calibration reference and CNM effects

The QGP medium is “transparent” to electroweak bosons, but in their production mechanisms, they are often coupled with a parton emitted in the opposite direction in azimuth and at similar p_T that can interact with the QGP medium. This is shown schematically for prompt photons in Fig 1.

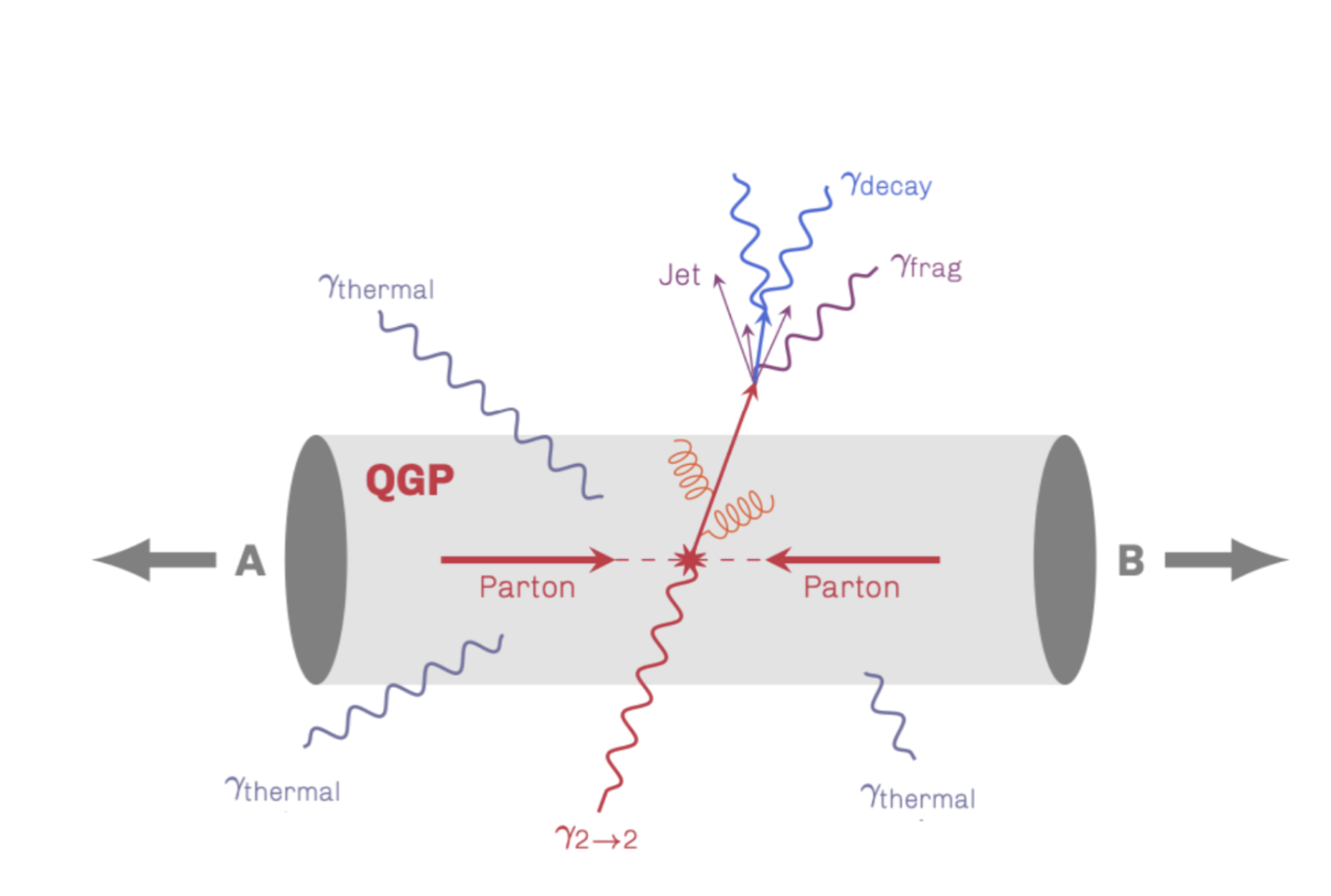
Figure 1: Schematical view of the production of the different photon sources in a heavy-ion collision, in particular, of a hard parton scattering with an outgoing prompt photon (2→2) correlated with an outgoing parton losing energy in the QGP.

Therefore, the measurement of electroweak bosons energy and angle with respect to the opposite side parton are relevant to “calibrate” medium effects affecting the associated parton. However, the production of electroweak bosons can still be affected due to cold nuclear matter (CNM) effects—primarily modifications of the parton distribution functions in nuclei (nPDF), such as shadowing at low Bjorken-x (the fraction of the momentum of the nucleon carried by each incoming parton), and isospin effects (colliding neutron or proton) [5,6,7]. We want to confirm experimentally in the study presented here the transparency of the QGP to photons and the CNM effects on their production from low to high transverse momentum (p_T).

Among the electroweak bosons, prompt photons are particularly interesting since: (i) they can be measured to relatively low p_T‘s (a few tenths of GeV/c), where QGP effects of the associated parton are larger, and CNM effects on the prompt photon production are stronger; and (ii) due to their higher production rate than other electroweak particles, although their measurement is more challenging than Z bosons due to the different dominant photon sources in the collision.

Prompt photons are produced directly by hard-scattering partons rather than by particle decays. At the leading order in perturbative QCD, prompt photons are produced via the processes: quark–gluon Compton scattering qg → qγ, and quark–antiquark annihilation qq → gγ and, with a much smaller contribution, qq → γγ. Higher-order processes involving parton fragmentation and bremsstrahlung also create prompt photons, “fragmentation photons”, whose production can be affected by jet quenching [7].

We are interested on the measurement of the photons from Compton scattering. Unfortunately, their measurement is challenging due to the dominance of other photon sources in high-energy hadronic collisions: the photon production yield is largely dominated by photons originating from neutral meson decays, one to two orders of magnitude more than prompt photons; and the fragmentation photons production, which is of similar magnitude to leading order prompt photons, although fragmentation photons are dominant below roughly 20 GeV/c [7].

Experimentally, a clean separation of the different prompt photon sources is difficult, but fragmentation and decay photons can be suppressed by selecting “isolated photons”. In Compton and annihilation processes no other particles are emitted in the direction of the photon, except for the collision underlying event. Decay and fragmentation photons are produced nearby other parton-jet particle fragments, and thus, they are “not isolated”.

After the isolation criteria discussed below are applied, the dominant process at the origin of the photon at the LHC are quark–gluon Compton scatterings, which are directly sensitive to the gluon density. The high √s of collisions at the LHC allow access to very small values of the longitudinal momentum fraction Bjorken x of the initial-state partons in the nucleons and nuclei, essentially gluons. The gluon PDF has a much larger uncertainty than the quark PDF. From the photon momentum one can extract the variable x_T = 2 p_T/√s, which is basically the Bjorken-x of the PDF in photon measurements at midrapidity (y~0). Therefore, isolated-photon measurements at the LHC allow the low-x gluon content of one of the incoming protons or nuclei to be probed, thus constraining the PDF and nPDF [7].

How prompt photons are selected? The isolation method

A photon is called “isolated” if the total transverse momentum of other particles found within a cone of radius R in (azimuth, pseudorapidity) space around the photon, called "isolation momentum", remains below a certain threshold. This requirement—applied in both experiments and theoretical calculations—helps reduce the contribution from fragmentation and decay photons, which usually come from hadrons produced in jets.

In Pb–Pb collisions at the LHC, an additional challenge arises from the large particle underlying event. In central collisions, its average density can reach roughly 150 GeV/(c rad). This contribution is subtracted from the isolation momentum by estimating collision-by-collision the p_T density looking to regions out of the isolation cone making sure the jet on the opposite side is avoided [9,10].

Building on the concept of isolated photons as a clean electromagnetic probe, the ALICE Collaboration has measured the p_T-differential cross section of isolated prompt photons in both pp and Pb–Pb collisions at √s_NN = 5.02 TeV [9,10]. Photons were considered isolated if the isolation momentum in a cone of radius R around the photon was below 1.5 GeV/c. Two cone sizes were used—R = 0.4 and R = 0.2—and measurements were performed for different centrality classes of Pb–Pb collisions (reflecting the impact parameter, or distance between the colliding nuclei). While R = 0.4 is commonly used in pp and proton–nucleus collisions because it captures most of the jet components, in nucleus–nucleus collisions a smaller radius (R = 0.2) helps mitigate large fluctuations from the underlying event when calculating isolation momentum, yet still contains the bulk of the jet core.

How the jet quenching is observed experimentally: The nuclear modification factor

The production mechanisms of hard probes are well known from quantum chromodynamics (QCD) theory in proton-proton (pp) collisions. Their interactions with the QGP can be studied by comparing how often these particles are produced (their “production cross section”) in nucleus–nucleus (AA) collisions versus proton–proton (pp) collisions, as a function of transverse momentum (p_T) and pseudorapidity (η). This comparison assumes that an AA collision can be viewed as a superposition of multiple nucleon–nucleon collisions, characterized by an average number of collisions. The cross-section modification is typically studied via the “nuclear modification factor” R_AA which derives from the following formula:

R_AA = 1/⟨N_coll⟩ (d²σ_AA/dp_Td𝜂) / (d²σ_pp/dp_Td𝜂)

for a given particle or jet (collimated spray of particles resulting from parton fragmentation in vacuum). Note that the ⟨N_coll⟩ depends strongly on the collision centrality (related to the impact parameter b, the distance between the centre of the colliding nuclei) [8]. In central collisions (with small b, around the 0–10% centrality range), ⟨N_coll⟩ can be about 1500, whereas in peripheral collisions (with large b, around 70–90% centrality), it drops to roughly 11.

But how does the QGP affect different types of particles? Partons interact strongly with the medium, while electroweak bosons do not, leading to different behaviours in their R_AA. Consequently, the nuclear modification factor R_AA for high-p_T particles and jets (partonic origin) is typically below 1, significantly below 1 for the more central collisions. On the other hand, electroweak bosons do not interact strongly with the QGP medium, escaping unmodified, implying that, in principle, their R_AA should be 1. This makes electroweak bosons relevant to calibrate the ⟨N_coll⟩ scaling and the medium effects on partons as discussed above. However, electroweak bosons can still exhibit moderate deviations from R_AA = 1 due to cold nuclear matter (CNM) effects.

Isolated photon cross section measurements in ALICE

The ALICE data were taken at mid-rapidity (|η| < 0.67) over a photon p_T range of roughly 10–14 GeV/c up to 40–140 GeV/c, with exact boundaries depending on the collision system, centrality class, and cone radius. This approach extends the measurement to lower photon p_T values compared to earlier ATLAS and CMS studies, which started around 20–25 GeV/c.

The p_T-differential cross section for R = 0.4 rad is shown in Fig. 2-left for measured data and NLO pQCD calculations. The calculations for Pb–Pb collisions use nPDF, and for pp collisions use PDF. The theory calculations are done without centrality selection (equivalent to a nucleon–nucleon collision) and are scaled by ⟨N_coll⟩ for each measured centrality class to compare to the data. Figure 1-right shows the ratio data over theory. One can appreciate the good agreement between data and theoretical calculations, particularly in the most central Pb–Pb collisions, which validate the theoretical description by incorporating CNM effects. Note that those effects are dominant below 20 GeV/c, which this measurement covers. The same degree of agreement is observed for R = 0.2 rad [8,9]. Furthermore, good agreement is observed in all centrality classes for Pb–Pb collisions, showcasing the validity of ⟨N_coll⟩ scaling.

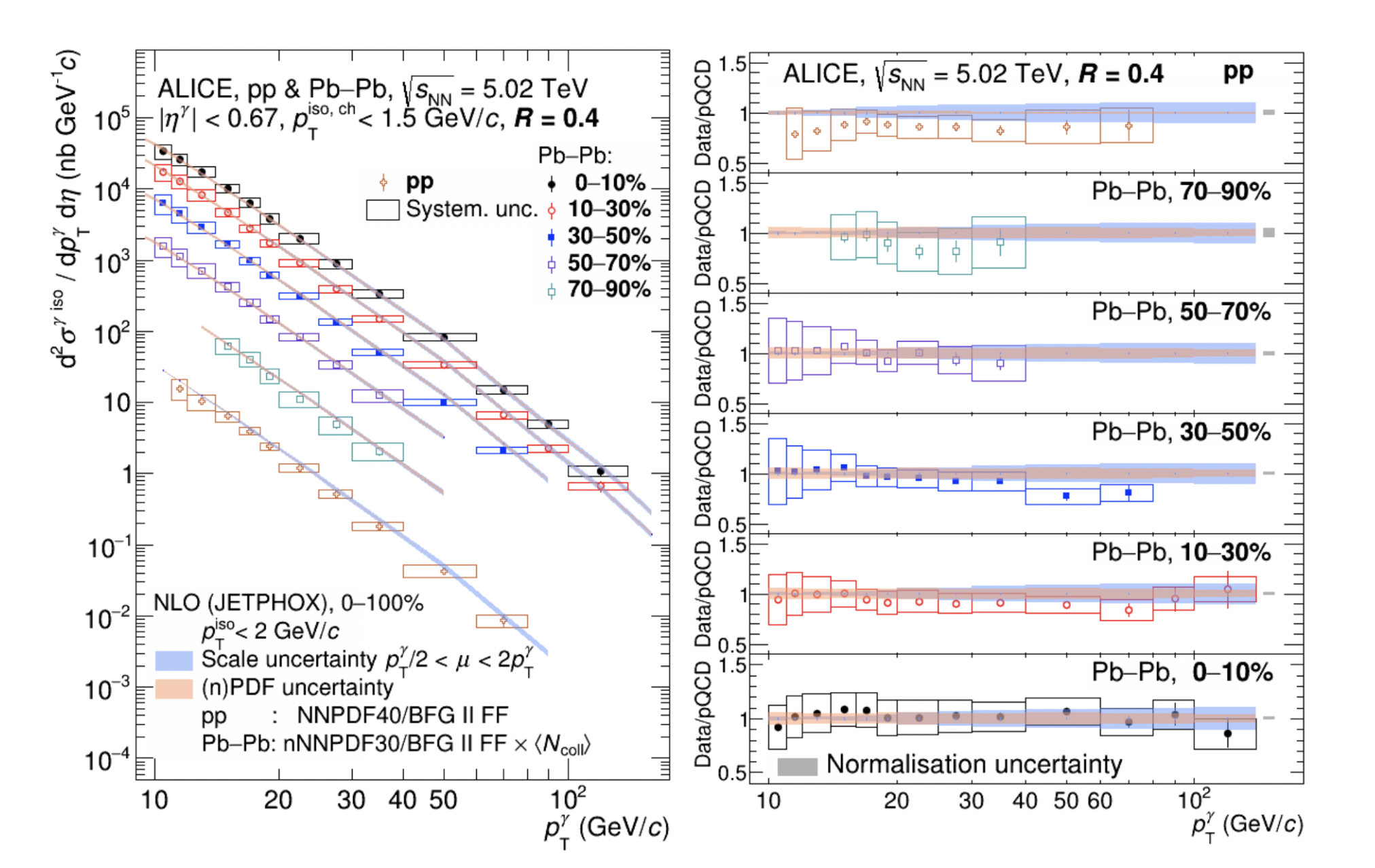
Figure 2: Left: Isolated-photon differential cross section measured in pp and Pb–Pb collisions at √s_NN = 5.02 TeV for five Pb–Pb centrality classes for R = 0.4. The bands correspond to NLO pQCD calculations with JETPHOX, for Pb–Pb collisions calculated for the 0–100% centrality class and scaled by ⟨N_coll⟩. Right: Ratio of data over JETPHOX NLO pQCD calculations. The bands centred at unity correspond to the JETPHOX pQCD calculations. From [8].

For the first time in central Pb–Pb collisions, the ALICE Collaboration has measured the ratio of cross sections obtained with different isolation radii (R = 0.4 and R = 0.2). This measurement is also performed in pp collisions at lower photon p_T than previous ATLAS results (the latter was for √s = 13 TeV and p_T > 250 GeV/c [11]). Figure 3 displays this ratio for central Pb—Pb collisions and pp collisions. This cross-section ratio is sensitive to QCD radiation because shrinking the isolation cone (while keeping the same isolation momentum threshold) excludes a portion of the jet fragments, effectively increasing the rate of photons produced via NLO pQCD fragmentation [12]. 

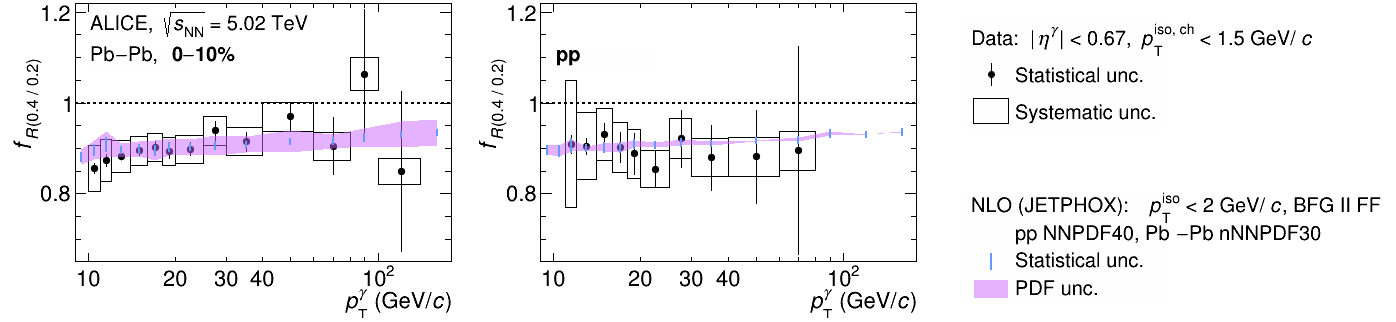
Ratio of isolated-photon cross section measured with R = 0.4 over R = 0.2 for Pb–Pb and pp collisions at √sNN = 5.02 TeV, for Pb–Pb collisions (left), and pp collisions (right). The violet band corresponds to pQCD calculations with JETPHOX. From [8].

Experimentally, the ratio ranges between 0.8 and 1 with no clear dependence on p_T. In contrast, NLO pQCD calculations predict a value around 0.9, which rises slightly at higher p_T; overall, the calculations describe the cone-size dependence well when an isolation criterion is included. These measurements can further constrain the non-perturbative part of the fragmentation function at lower p_T once experimental uncertainties are reduced in future studies. Interestingly, the ratios observed in pp and Pb–Pb collisions (for various centrality classes) are consistent with one another—no centrality-dependent modification is evident in Pb–Pb collisions. This is somewhat surprising, as one might expect fragmentation photons to be suppressed in the dense QGP medium.

Figures 4 and 5 show the isolated-photon R_AA, as a function of p_T and five Pb–Pb centrality classes measured for the cone radii R = 0.4 rad in Fig. 3, and as a function of centrality for 20 < p_T < 25 GeV/c and R = 0.2 and 0.4 rad in Fig 4. Figure 4 also shows the integrated p_T Z⁰ bosons R_AA measured by the ATLAS [15] and CMS [16] Collaborations. The isolated photon and Z⁰-boson R_AA are generally compatible with unity, further validating the ⟨N_coll⟩ scaling. The isolated-photon measurement in Fig. 4 agrees with the CMS measurement for p_T > 25 GeV/c. The strong suppression observed for high-p_T hadrons in central Pb–Pb collisions with respect to pp collisions, shown in Fig. 5 (right) for 8 < p_T < 20 GeV/c, which is due to the jet-quenching in the QGP, is therefore not observed for isolated photons and Z⁰ bosons, as expected.

For the most peripheral class (70–90%), the ratio shows a tendency to fall below 1. By comparison, in more central collisions, charged-hadron R_AA is lower than unity, but in peripheral collisions, it is closer to unity—though still unexpectedly low at around 0.75. A similar trend was previously observed at RHIC in Au–Au and d–Au collisions at √s_NN = 200 GeV. It can be attributed to biases in selecting centrality and collision geometry, which the Glauber model does not fully capture [14]. Indeed, in the 50–90% and 70–90% centrality classes, the HG-PYTHIA model [14] predicts an R_AA bias of about 0.91 and 0.82 (red line in the figures), respectively, which agrees well with the measured isolated-photon and CMS Z⁰-boson R_AA, as Fig. 5 shows. However, ATLAS measurement of the Z⁰-boson R_AA in peripheral collisions is significantly above unity. A possible explanation for this different behaviour is given in Ref. [17].

The R_AA from the JETPHOX NLO pQCD calculations for 0–100% centrality is also shown in Fig. 3 for R = 0.4; for R = 0.2, it is almost identical. Like the data, the NLO pQCD R_AA is close to unity for p_T > 40–50 GeV/c. In contrast, a moderate suppression is expected at lower p_T (≈ 0.8 at p_T = 10 GeV/c for 0–100% centrality), which can be attributed to CNM differences in the proton and nucleus PDFs, but still compatible with ALICE data within uncertainties. The particularly good agreement of the Pb–Pb collisions data with NLO pQCD + nPDF in Figs. 2 suggests that the data support the R_AA calculated with this NLO pQCD framework.

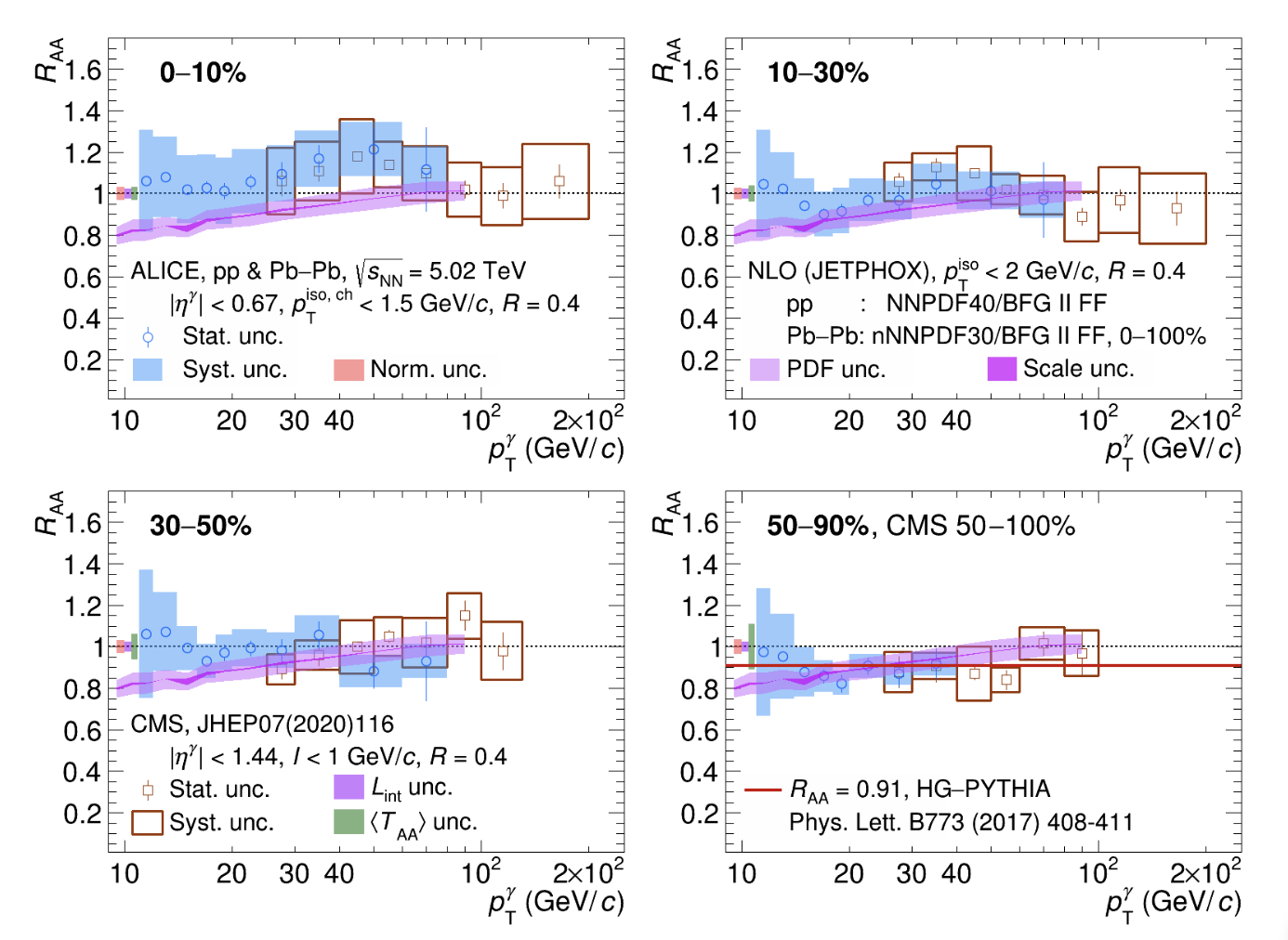
Figure 4: Nuclear modification factor R_AA for isolated photons measured by ALICE [9,10] and CMS [13] for isolation-cone radius R = 0.4 at √s_NN = 5.02 TeV and for four centrality classes. The solid line in the peripheral centrality class 50–90% at R_AA = 0.91, is the result of the HG-PYTHIA model [14]. The bands correspond to pQCD calculations with JETPHOX for Pb–Pb collisions (nPDF) for 0–100% centrality over pp collisions (PDF).

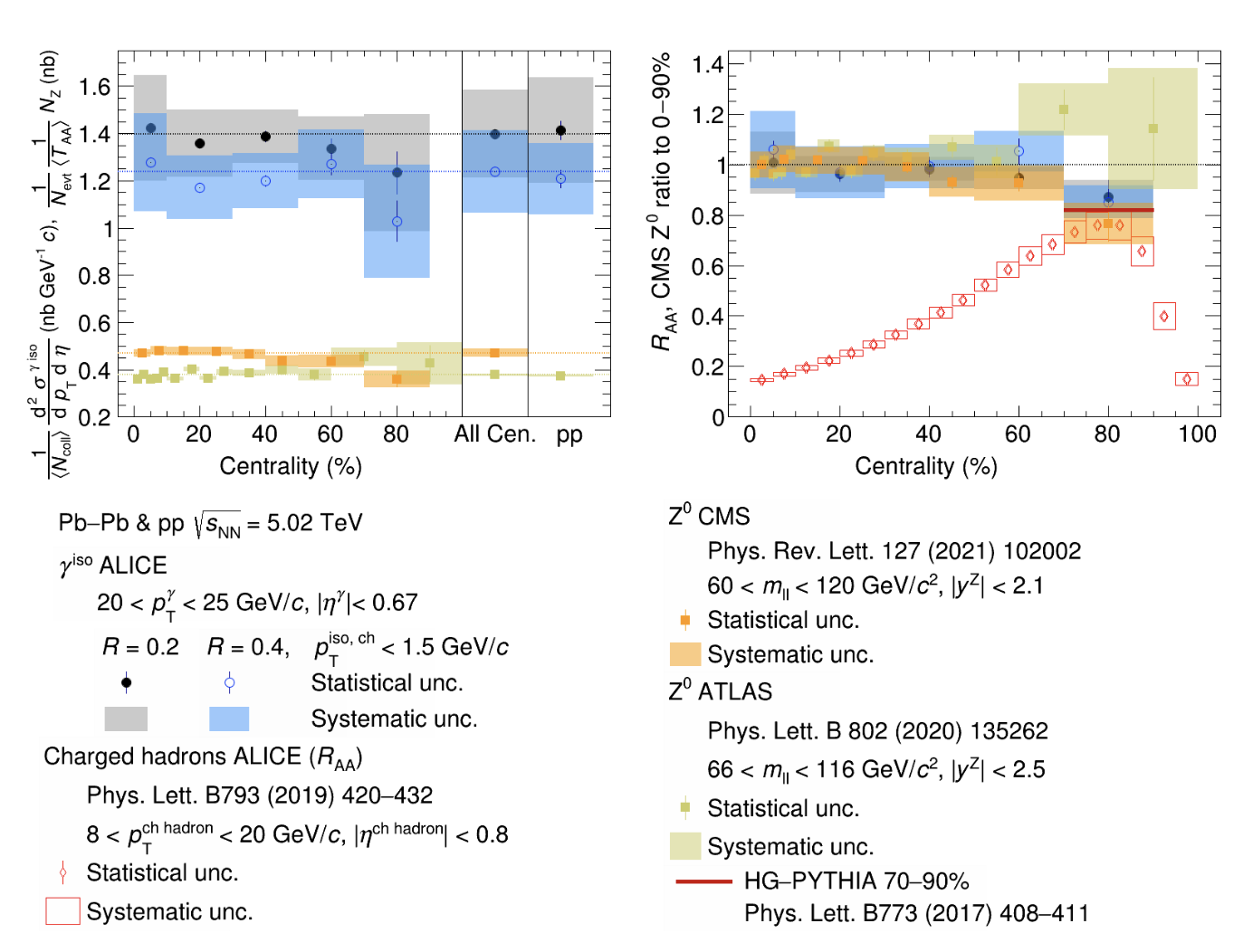
Figure 5: Left: Cross section of isolated photons with 20 < p_T < 25 GeV/c for R = 0.2 and 0.4 as a function of the centrality class or collision system in Pb–Pb and pp collisions at √s_NN = 5.02 TeV. Also shown is the p_T-integrated Z⁰ bosons cross section measured by CMS [16] for Pb–Pb collisions at √s_NN = 5.02 TeV and ATLAS [15] for Pb–Pb collisions at √s_NN = 5.02 TeV. The 0–90% centrality class for isolated photons is calculated as the ⟨N_coll⟩-weighted average of the other centrality classes. The dotted lines indicate the value of the ALICE 0–90% centrality class centre. Right: R_AA for ALICE isolated photons and ATLAS Z⁰ bosons, and the ratio of the CMS Z⁰ boson cross sections in each centrality class to the one in 0–90%. The solid line in peripheral centrality class 70–90% at R_AA = 0.82 corresponds to the expectation from the HG-PYTHIA model [14]. The Z⁰ scale made with the nuclear overlap function ⟨T_AA⟩ = ⟨N_coll⟩/σ_NN-INEL, with σ_NN-INEL the NN inelastic cross section.

In summary, the measured isolated-photon R_AA in different centrality classes are consistent with unity and with the theoretical predictions from low to high p_T. This indicates that: first, isolated photons are not affected by the quark–gluon plasma; second, data agree with predictions incorporating initial state nuclear effects; and third, precise measurements of electroweak bosons serve as a reference to validate the ⟨N_coll⟩ scaling, in particular, in peripheral collisions.

The precision of the current measurements does not set strong constraints on the models, but the combination of the higher luminosity LHC Run 3 and 4 taken with ALICE, with an upgraded detector that currently takes the full luminosity delivered by the LHC, will reduce significantly the uncertainties, in particular in the pp reference system and peripheral Pb–Pb.

Another point to consider is the potential of isolated photons and electroweak bosons in general to study the biases introduced in the measurements of small collision systems (pp, p–Pb) when doing studies as a function of the collision particle multiplicity or event classification parameters (spherocity, flatenicity, etc). In such systems, surprising effects that could be attributed to the presence of the QGP have been observed when studying very high multiplicities. It is, therefore, important to discard biases like the ones observed in peripheral Pb–Pb collisions with well-calibrated probes such as isolated photons. Such measurements are statistics-hungry and must be considered with Run 3 and 4 data.

References

[1] ALICE Collaboration, S. Acharia, “The ALICE experiment – A journey through QCD”, Eur. Phys. J. C 84 (2024) 813, arXiv:2211.04384 [nucl-ex].

[2] B. V. Jacak and B. Muller, “The exploration of hot nuclear matter”, Science 337 (2012) 310–314.

[3] J. D. Bjorken, “Energy loss of energetic partons in quark-gluon plasma: Possible extinction of high p_T jets in hadron-hadron collisions”, Fermilab report FERMILAB-PUB-82-059-T, 1982. https://lss.fnal.gov/archive/1982/pub/Pub-82-059-T.pdf.

[4] D. d’Enterria, “Jet quenching”, in Relativistic Heavy Ion Physics, R. Stock, ed., vol. 23, p. 99. Springer-Verlag Berlin Heidelberg, 2010. arXiv:0902.2011 [nucl-ex].

[5] ATLAS Collaboration, G. Aad et al., “Centrality, rapidity and transverse momentum dependence of isolated prompt photon production in lead-lead collisions at √s_NN = 2.76 TeV measured with the ATLAS detector”, Phys. Rev. C 93 (2016) 034914, arXiv:1506.08552 [hep-ex].

[6] B.-W. Zhang and I. Vitev, “Direct photon production in d+A and A+A collisions at RHIC”, Phys. Lett. A 24 (2009) 2649–2658, arXiv:0810.3194 [nucl-th].

[7] D. d’Enterria and J. Rojo, “Quantitative constraints on the gluon distribution function in the proton from collider isolated-photon data”, Nucl. Phys. B 860 (2012) 311–338, arXiv:1202.1762 [hep-ph].

[8] ALICE Collaboration, B. Abelev et al., “Centrality determination of Pb–Pb collisions at √s_NN = 2.76 TeV with ALICE”, Phys. Rev. C 88 (2013) 044909, arXiv:1301.4361 [nucl-ex].

[9] ALICE Collaboration, S. Acharia, “Measurement of the inclusive isolated-photon production cross section in pp and Pb–Pb collisions at √s_NN = 5.02 TeV.” arXiv:2409.12641 [nucl-ex].

[10] ALICE Collaboration, “Supplemental figures: Measurement of the inclusive isolated-photon production cross section in pp and Pb–Pb collisions at √s_NN = 5.02 TeV.” ALICE-PUBLIC-2024-003, 2024. https://cds.cern.ch/record/2910556.

[11] ATLAS Collaboration, G. Aad et al., “Inclusive-photon production and its dependence on photon isolation in pp collisions at √s = 13 TeV using 139 fb⁻¹ of ATLAS data”, JHEP 07 (2023) 86, arXiv:2302.00510 [hep-ex].

[12] X. Chen, T. Gehrmann, N. Glover, M. Höfer, and A. Huss, “Isolated photon and photon+jet production at NNLO QCD accuracy”, JHEP 04 (2020) 166, arXiv:1904.01044 [hep-ph].

[13] CMS Collaboration, A. M. Sirunyan et al., “The production of isolated photons in PbPb and pp collisions at √s_NN = 5.02 TeV”, JHEP 07 (2020) 116, arXiv:2003.12797 [hep-ex].

[14] C. Loizides and A. Morsch, “Absence of jet quenching in peripheral nucleus–nucleus collisions”, Phys. Lett. B 773 (2017) 408–411, arXiv:1705.08856 [nucl-ex].

[15] ATLAS Collaboration, G. Aad et al., “Z boson production in Pb+Pb collisions at √s_NN = 5.02 TeV measured by the ATLAS experiment”, Phys. Lett. B 802 (2020) 135262, arXiv:1910.13396 [nucl-ex].

[16] CMS Collaboration, A. M. Sirunyan et al., “Constraints on the Initial State of Pb–Pb Collisions via Measurements of Z-Boson Yields and Azimuthal Anisotropy at √s_NN = 5.02 TeV”, Phys. Rev. Lett. 127 (2021) 102002, arXiv:2103.14089 [hep-ex].

[17] F. Jonas and C. Loizides, “Centrality dependence of electroweak boson production in PbPb collisions at the CERN Large Hadron Collider”, Phys. Rev. C 104 (2021) 044905