The potential of a post-LHC collider to explore for hints of new physics relies on technological advancements but also on theoretical developments that will allow very accurate background determinations. With a concensus growing for a lepton collider offering a wide range of precision Higgs and electroweak measurements it is important to consider the required theoretical improvements. First, and foremost there is a clear need to increase the accuracy of automated computations of inclusive quantities from the first order next-to-leading order (NLO) to the next-to-next-to-learing order (NNLO). As stated in the Physics Briefing Book of the EPPSU: "Fully automated NLO tools are now available and the next challenge is to upgrade them to the NNLO level". The article discusses recent progresses and the challenges lying ahead to progress in parallel with advancements in the accelerator and experimental frontier. 

The quest to understand the constituents and forces of Nature, from the shortest accessible distances to processes at astrophysical scales, has led physicists to build the Standard Model of particle physics, now celebrating its jubilee. It has a long record of success in quantitatively describing all experimental results and precisely predicting a wide variety of new phenomena.

High-energy particle colliders have been extremely powerful and successful tools, furnishing copious data that have yielded discoveries of new particles and precision measurements of fundamental interaction parameters. The energy frontier is currently at the CERN’s Large Hadron Collider (LHC), which today hosts the most important infrastructure in particle physics not only in Europe but worldwide. The celebrated 2012 discovery of the Higgs boson was made there by the scientific teams of the two general-purpose detectors, ATLAS and CMS. The LHC has just come to the end of its second run. With the end of this run, the current era of broad searches is drawing to a close.

A new era of precision measurements is dawning with Run 3 and the subsequent High Luminosity LHC Run. Precision measurements will require precise theoretical predictions if the scientific community is to fully exploit the machine’s potential, as well as the work of its thousands of experienced experimenters, early-stage researchers, and technicians. A new effort to produce theoretical predictions at the level of precision needed for upcoming data is thus most timely. This effort will also lay the groundwork for a theoretical program to accompany not only LHC experiments through their planned schedule over the next decade and a half but also the long-run scientific program of the high-energy physics community, as for instance the Future Circular Collider (FCC) project. 

The theoretical prediction of observable distributions can be carried out within the well-understood framework of perturbative QCD [1]. The core ingredients are scattering probabilities, i.e. cross sections and differential distributions. They can be determined by taking into account (i.e. integrating and summing) all possible configurations of allowed final states, and all configurations of the gluon and quark beams which the protons are effectively providing. Quantum fluctuations give rise to ‘virtual’ corrections to these scattering probabilities. Moreover, states that differ by additional massless particles — such as a photon in quantum electrodynamics or a gluon in QCD — of too low energy or too nearby in angle, are indistinguishable from each other. We need to integrate therefore over all such contributions as part of the ‘real emission’ corrections. This integral, done naively, yields an infinite result, because the probability of finding such low-energy particles grows inversely with the decreasing energy (and likewise for the angle). These infinities are called infrared divergences. The divergences must be isolated and regulated. They can then be integrated, and the potentially divergent parts cancel against those arising from ‘virtual’ contributions. The remaining terms are finite and can be evaluated numerically.

It is necessary to stress that the first three orders of the expansion in the coupling constants, most importantly in the strong coupling, are especially relevant in the analysis of physical observables. Indeed, the leading order (LO) provides a rough estimate only, where neither the normalization (for instance the total fiducial cross section) nor the estimation of the theoretical uncertainty are reliable, due to the strong dependence on the unphysical renormalization and factorization scales. The next-to-leading order (NLO) provides a meaningful normalization, but it is only at the next-to-next-to-leading order (NNLO) that a reliable estimate of the precision of the theory predictions as well can be achieved. Besides, for many processes, the corrections turn out to be so large, that even higher loop calculations (NNNLO) are required [2].

At the first quantitative order in the perturbative expansion, the next-to-leading order (NLO), several approaches to the ‘real emission’ corrections have been developed, tested, and well understood [3]. As far as the ‘virtual’ corrections to the scattering amplitudes are concerned, our understanding has been impressively evolved over the last fifteen years. Thanks to the reduction of one-loop amplitudes to a set of Master Integrals (a minimal set of Feynman Integrals that form a basis of them [4]), either using unitarity methods [5] or an integrand-level approach [6], the way one-loop calculations are preformed has drastically changed, resulting in many fully automated numerical tools [7-11] (for a review on the topic see [12]), making the next-to-leading order (NLO) approximation the default precision for theoretical predictions at the LHC.

It is almost seventy years from the time Feynman Integrals were first introduced [13] and forty-five years since the dimensional regularisation [14] set up the framework for an efficient use of loop integrals in computing scattering matrix elements, and still the frontier of multi-scale multi-loop integral calculations (maximal both in number of scales and number of loops) is determined by the planar five-point two-loop massless integrals [15-16], recently computed. The reason for this slow pace is to be traced back to our unsatisfactory understanding of the structure of Feynman integrals and of the scattering amplitudes in general, and it is not just a matter of computing resources.

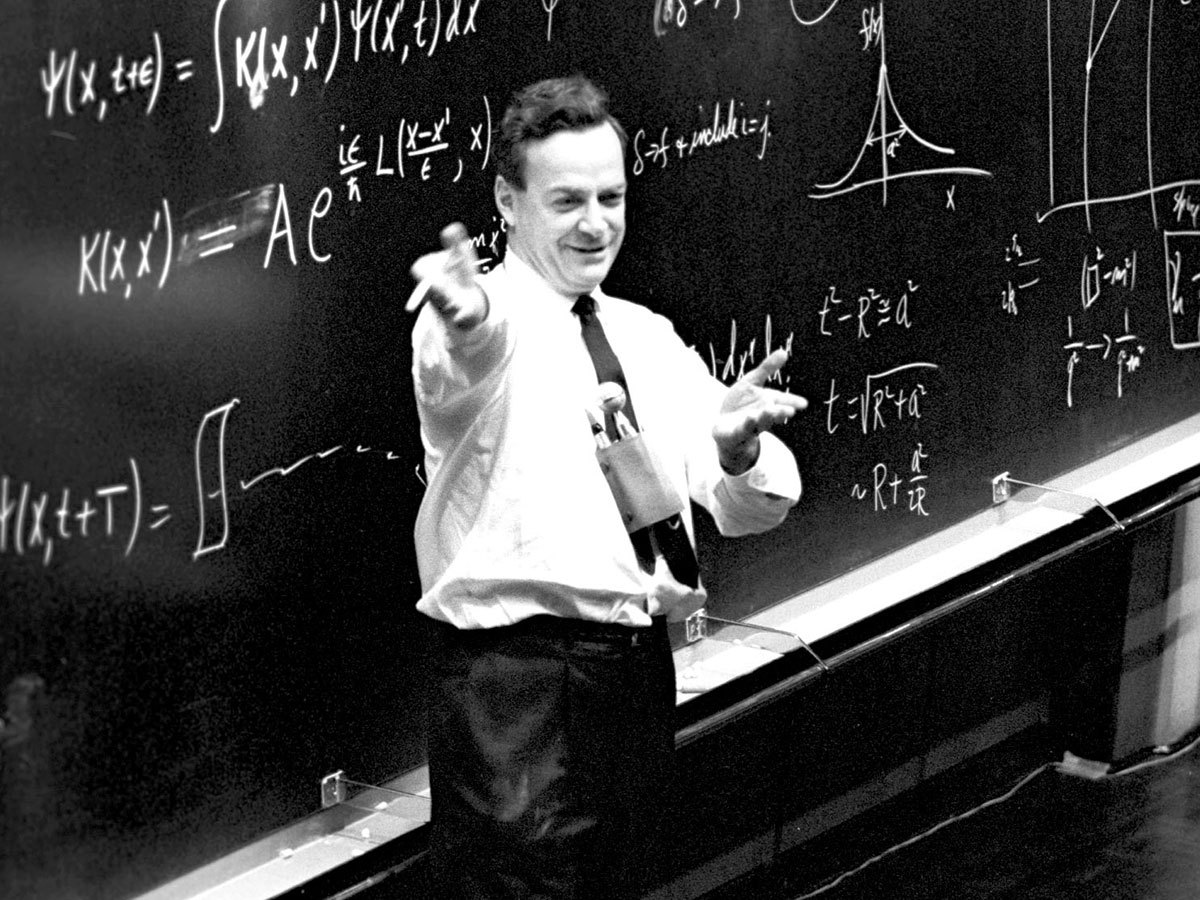
Richard Feynman in September 1949 published its two papers introducing the use the so-called Feynman diagrams greatly reducing the amount of computationsl involved in calcuating a rate or cross-section of a physics process. (Credits: CERN). 

Adding a new ‘next’ (NLO, NNLO, etc.) in the perturbative series is not just a technicality, but we are, unfortunately, entering a new unexplored territory, where new concepts and approaches have to be invented. We have therefore no other alternative but to explore the next ‘next’ order, with the aim at the ‘practical’ side, to provide more advanced theoretical predictions that will match the foreseen experimental precision, and at the ‘theoretical’ side, to unveil the structure of scattering amplitudes. 

The first published Feynman diagram (right) shows the classic case of two electrons exchanging a virtual photon. Such diagrams are essential in particle physics, as they represent terms in the equations, as well as illustrating particle interactions schematically. 

Calculations of multi-loop integrals have a long history. Contrary to the one-loop case, where Master Integrals have been known for a long time ago [17], a complete library of Master Integrals at two loops (or even beyond) is still missing. The overall most successful method to calculate multi-loop Feynman integrals is based on expressing them in terms of an integral representation over the so-called Feynman or Schwinger parameters. In fact, the introduction of the sector decomposition method [18-19], resulted in a powerful computational framework for their numerical evaluation [20]. Nevertheless, the most successful method to obtain analytic expressions and numerical estimates of multi-scale multi-loop Feynman Integrals is, for the time being, the differential equations approach [21]. With the introduction of the canonical form of the differential equations [22], a major step towards the understanding of the mathematical structure (multiple polylogarithms) of Feynman Integrals and subsequently of the scattering amplitudes has been achieved.

This paved the road for the computation of practically all two-loop QCD virtual amplitudes with massless internal states. Two-loop amplitude calculations with massive internal/external states [23] (relevant for instance to the top-pair production and to the mixed QCD and electroweak corrections) are also available and new mathematical structures (elliptic polylogarithms) have been developed in order to obtain analytic insight [24] of certain Feynman Integrals. At the same time, the calculation of ‘real emission’ corrections at the next-to-next-to-leading order, NNLO, which relies on the single– and doubly–unresolved parton contributions, seems to approach a certain state of maturity. The past few years we have seen remarkable developments in the understanding and the treatment of infrared singularities in NNLO computations, and a range of methods based on different physical ideas have been successfully developed and applied [25-33]. It is fair to say that, with the achieved completion of the vast majority of 2 → 2 scattering processes calculations, we have witnessed the start of the NNLO revolution.

Nevertheless, in order to reach the same level of understanding as in the NLO case, much more needs to be done. The current frontier of NNLO calculations is as always split in two directions. In the frontier of ‘real emission’ corrections, we seek to improve and automate the current computational tools and algorithms, with the aim to extent our NNLO calculations beyond the 2 → 2 barrier. On the other hand, with respect to the two-loop amplitudes, significant progress has been seen over the last couple of years. Two-loop amplitude reduction for 2 --> 3 processes is now well developed [34-36], even for massive external states [37]. Analytic expressions for the previously unknown two-loop five-point Master Integrals have also been obtained [15-16]. It is hoped that in the near future, not only theoretical predictions for 2 → 3 processes, as for instance three-jet, H+2jets or V+2jets production will be available, but also the groundwork for the extension and automation for arbitrary 2 → n processes will be established, marking the maturity era of the NNLO revolution.      

The developments and challenges described in this article call for further theoretical research, in order to promote our understanding of the makings of the Standard Model of particles physics and unveil, by comparing with the experimental measurements, the boundaries where new physics may lie.

References

[1] J.C.Collins, D.E.Soper and G.F.Sterman, Adv. Ser. Direct. High Energy Phys.  5 (1989) 1

[2] C.Anastasiou, C.Duhr, F.Dulat, F.Herzog and B.Mistlberger, Phys. Rev. Lett.  114 (2015) 212001

[3] S. Catani, M.H. Seymour, Nucl.Phys. B485 (1997) 291-419; S. Frixione, Z. Kunszt, A. Signer, Nucl.Phys. B467 (1996) 399-442; Zoltan Nagy, Davison E. Soper, JHEP 0309 (2003) 055

[4] G. Passarino, M.J.G. Veltman, Nucl. Phys. B160 (1979) 151-207; K. Chetyrkin and F. Tkachov, Nucl.Phys. B192 (1981) 159–204

[5] C. F. Berger, Z. Bern, L. J. Dixon, F. Febres Cordero, D. Forde, H. Ita et al., Phys. Rev. D78 (2008) 036003

[6] G. Ossola, C. G. Papadopoulos and R. Pittau, Nucl.Phys. B763 (2007) 147–169

[7] G.Bevilacqua, M.Czakon, M.V.Garzelli, A.van Hameren, A.Kardos, C.G.Papadopoulos, R.Pittau and M.Worek, Comput. Phys. Commun.  184 (2013) 986

[8] J.Alwall et al., JHEP  1407 (2014) 079

[9] F.Cascioli, P.Maierhofer and S.Pozzorini, Phys. Rev. Lett.  108 (2012) 111601

[10] G. Cullen, N. Greiner, G. Heinrich, G. Luisoni, P. Mastrolia, G. Ossola, T. Reiter and F. Tramontano, Eur. Phys. J. C 72 (2012) 1889

[11] S.Actis, A.Denner, L.Hofer, A.Scharf and S.Uccirati, JHEP 1304 (2013) 037

[12] R. K. Ellis, Z. Kunszt, K. Melnikov and G. Zanderighi, Phys.Rept. 518 (2012) 141–250

[13] R.P.Feynman, Phys. Rev. 76 (1949) 769.

[14] G.'t Hooft and M.J.G.Veltman, Nucl. Phys. B44 (1972) 189.

[15] C.G.Papadopoulos, D.Tommasini and C.Wever, JHEP  1604 (2016) 078

[16] D.Chicherin, T.Gehrmann, J.M.Henn, P.Wasser, Y.Zhang and S.Zoia, Phys. Rev. Lett. 123 (2019) no.4, 041603

[17] G. ’t Hooft and M. Veltman, Scalar One Loop Integrals, Nucl.Phys. B153 (1979) 365–401

[18] T. Binoth and G. Heinrich, Nucl. Phys. B585 (2000) 741

[19] C. Bogner and S. Weinzierl, Comput. Phys. Commun. 178 (2008) 596–610

[20] S. Borowka, G. Heinrich, S. Jahn, S. P. Jones, M. Kerner, J. Schlenk and T. Zirke, Comput. Phys. Commun.  222 (2018) 313

[21] A.V. Kotikov, Phys.Lett. B254 (1991) 158-164; T.Gehrmann and E.Remiddi, Nucl. Phys. B 580 (2000) 485

[22] J.M.Henn, Phys. Rev. Lett.   110 (2013) 251601

[23] M.Czakon, P.Fiedler and A.Mitov, Phys. Rev. Lett.  110 (2013) 252004

[24] J.Broedel, C.Duhr, F.Dulat and L.Tancredi, JHEP 1805 (2018) 093

[25] Anastasiou, C., Melnikov, K., and Petriello, F. (2004) Phys.Rev. D69, 076010.

[26] A. Gehrmann-De Ridder, T. Gehrmann and M. Ritzmann, JHEP 1210 (2012) 047

[27] S. Catani and M. Grazzini, Phys. Rev. Lett. 98 (2007) 222002

[28] P. Bolzoni, G. Somogyi and Z. Trocsanyi, JHEP 1101 (2011) 059

[29] M. Czakon and D. Heymes, Nucl. Phys. B 890 (2014) 152

[30] R. Boughezal, C. Focke, X. Liu and F. Petriello, Phys. Rev. Lett. 115 (2015) no.6, 062002

[31] M. Cacciari, F. A. Dreyer, A. Karlberg, G. P. Salam and G. Zanderighi, Phys. Rev. Lett. 115 (2015) no.8, 082002

[32] F. Caola, K. Melnikov and R. Rontsch, Eur. Phys. J. C 77, no. 4, 248 (2017)

[33] L. Magnea, E. Maina, G. Pelliccioli, C. Signorile-Signorile, P. Torrielli and S. Uccirati, JHEP 1812 (2018) 107

[34] S.Badger, C.Brønnum-Hansen, H.B.Hartanto and T.Peraro, Phys. Rev. Lett. 120 (2018) no.9, 092001

[35] S.Badger et al., Phys. Rev. Lett.   123, no. 7, 071601 (2019)

[36] S.Abreu, J.Dormans, F.Febres Cordero, H.Ita, B.Page and V.Sotnikov, JHEP  1905 (2019) 084

[37] H.B.Hartanto, S.Badger, C.Brønnum-Hansen and T.Peraro, arXiv:1906.11862 [hep-ph]

Image note: In 1972, the 1st Europhysics Conference on Neutrinos (Neutrino'72) was held in this town, gathering several major physicists among whom Richard Feynman. A memorial tree was planted for that occasion by Feynman in the Tagore Promenade, which is still standing today among many other trees by famous poets, politicians and scientists (Credits:https://indico.ific.uv.es/event/3356/page/58-venue).