The Baryon Antibaryon Symmetry Experiment (BASE) is recognized for performing the most precise measurements of the fundamental properties of antiprotons and protons, such as the 16 p.p.t. precision measurement of the antiproton-to-proton charge-to-mass ratio [1], the 1.5 p.p.b. precision measurement of the antiproton magnetic moment [2], and the 300 p.p.t. measurement of the proton magnetic moment [3]. These measurements are conducted in a cryogenic multi-Penning trap system using single particles [4, 5]. Particles in Penning traps have a well-defined motion described by the combination of three harmonic oscillatory modes: the magnetron motion at about 10 kHz, the axial motion at 600 kHz, and the modified cyclotron motion at 29 MHz. Specially designed cryogenic superconducting resonators [6] allow for non-destructive detection of these frequencies, providing information about the fundamental properties of trapped particles. The precision of the measurements strongly depends on the amplitudes of the oscillations, as larger amplitudes increase the influence of inhomogeneities and imperfections of the trap on the measured frequency, and also induce heating rates [7]. Therefore—in particular in quantum-resolved measurements—it is crucial to reduce the oscillation amplitudes, a process we refer to as cooling. Recently, BASE reported two major improvements in cooling of single (anti)protons: one using the new Cooling Trap technique implemented at BASE-CERN [8], and another using Sympathetic Cooling via Image Currents at BASE-Mainz [9].

In the "Cooling Trap" manuscript [8], BASE reports on the implementation of a new Penning trap system optimized for sub-thermal cooling of the cyclotron mode using a Maxwell-demon protocol. The new device consists of two stacked Penning traps: a Cooling Trap (CT) and an Analysis Trap (AT), as shown in Figure 1. During the cooling procedure, the particle is first placed in the AT, where its cyclotron energy is measured with a resolution of 0.86 μeV in just 10 seconds, thanks to the strong magnetic inhomogeneity of 266(8) kT/m². The particle is then transported to the CT, where it couples to a connected high-Q resonant circuit, which acts as a very efficient cooling resistor. A special trap design, combined with sophisticated electronics, allows for a cooling time constant of 4.5 seconds, more than 10 times faster than in previous state-of-the-art experiments. The particle’s temperature probes the Boltzmann distribution, with a temperature of 4.1(2) K defined by the resonant circuit. After thermalization, the particle is sent back to the AT, where its cyclotron energy is measured again. In this procedure, BASE was able to cool the cyclotron energy of a single antiproton below 200 mK in just 8 minutes, improving the total cooling time by more than a factor of 100 compared to earlier experiments.

Achieving these ultra-low cyclotron temperatures is crucial for BASE to perform Non-destructive Spin Quantum Transition Spectroscopy with a single antiproton. The improvements in the analysis trap electronics, combined with fast cooling, reduced the antiproton spin state identification error rate from 7.9% [10] to below 0.023%, providing essentially error-free measurements. This achievement has an enormous impact on high-precision comparisons of the fundamental properties of protons and antiprotons, as well as on magnetic moment measurements of heavier nuclei, such as ³He²⁺, deuteron, and antideuteron.

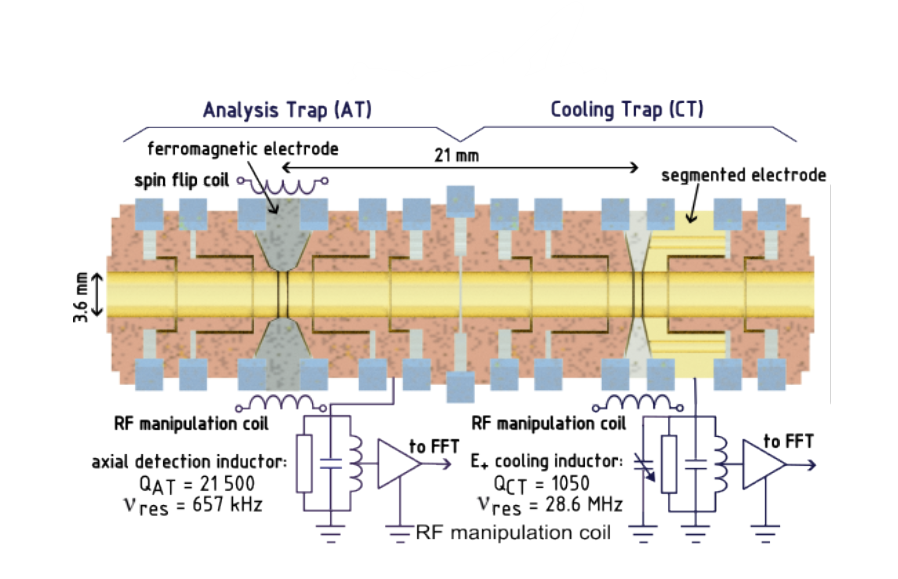
Figure 1: The experimental setup of the BASE experiment used for cooling of the cyclotron motion of a single antiproton which consists of two stacked Penning traps: a Cooling Trap (CT) and an Analysis Trap (AT), each connected to respective electronic circuits.

While the cooling trap offers extraordinary performance and is the key device for next-generation measurements, it is also near the technological optimum, so that for even lower temperatures at shorter times new techniques are required. This is why the BASE subgroup at the University of Mainz, Germany, is currently developing a special kind of sympathetic cooling technique. Usually, sympathetic cooling refers to the mixing of a target particle with colder particles in the same trapping potential, so that the target particle is cooled via Coulomb interaction. However, the target particle being antimatter severely complicates existing techniques. As a result, we place the target ion and laser-coolable ions, which serve as a cold bath, in distinct traps (Figure 2). The coupling of the ions is realized via their image currents, which they induce in the trap electrodes due to their oscillatory motion. These image currents are “collected” by a capacitor, which builds up a voltage signal and then in turn affects the ions’ motions in both traps. This is the exact electrical equivalent to putting a spring in between two pendulums. However, these image currents are tiny, on the order of fA. To enhance the coupling strength, we shim the capacitance with a coil, thus effectively composing a parallel RLC circuit. In a simple picture, the ions’ tiny image currents are enhanced by the resonance condition of the RLC circuit. In 2021, BASE was able to demonstrate image-current-mediated sympathetic cooling for the very first time [11]. Now, we further improved our hardware setup [12] and refined our understanding of the cooling process [13]. As a result, we were recently able to lower the axial temperature to about 170 mK [9], which is 15 times better than their previous record and a factor 50 below the environmental temperature.

In summary, the technological innovations of both methods will greatly influence the precision of future measurements with single (anti)protons. Moreover, the developments described here can be applied to any species of particles, giving both methods very broad potential applications.

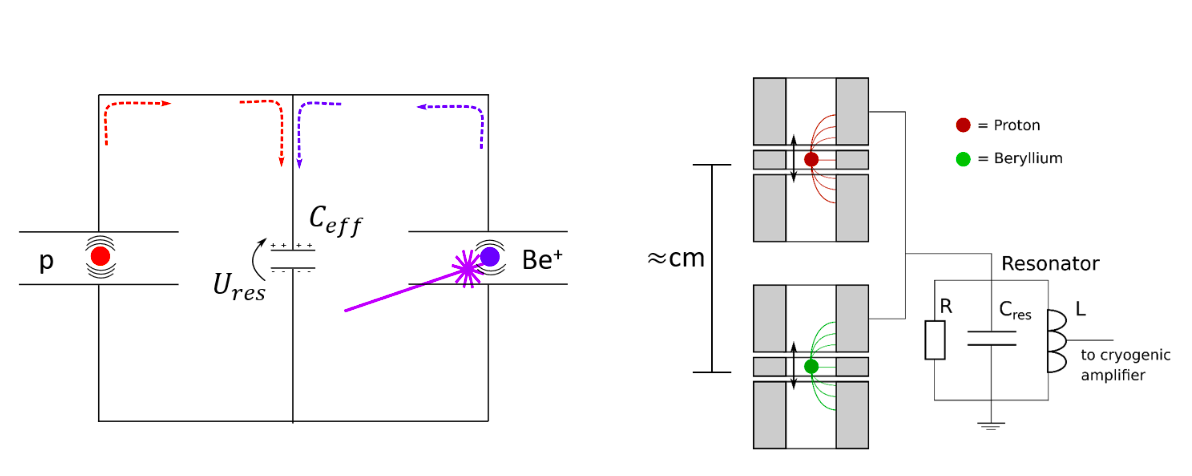
Figure 2 :  Schematic of the experimental setup: A cloud of beryllium ions is trapped in one Penning trap, and a single proton is trapped in the second one. Both traps are coupled via a common resonator.

Further Reading

[1] S. Ulmer, et al. Nature 601.7891 (2022): 53-57.

[2] C. Smorra, et al. Nature 550.7676 (2017): 371-374.

[3] G. Schneider, et al. Science 358.6366 (2017): 1081-1084.

[4] B.M. Latacz and S. Ulmer, et al. Eur. Phys. J. D 77.6 (2023): 94.

[5] C. Smorra and S. Ulmer, et al. Eur Phys J Spec Top 224 (2015): 3055-3108.

[6] H. Nagahama, et al. Rev. Sci. Instrum. 87.11 (2016).

[7] M.J. Borchert, S. Ulmer, et al. Phys. Rev. Lett. 122.4 (2019): 043201.

[8] B.M. Latacz, M. Fleck, S. Ulmer, et al. Phys. Rev. Lett. 133.5 (2024): 053201.

[9] C. Will, M. Wiesinger, P. Micke, et al. Phys. Rev. Lett. 133, 023002.

[10] C. Smorra and S. Ulmer, et al. Phys. Rev. Lett. 769 (2017): 1-6.

[11] M. Bohman, et al. Nature 596.7873 (2021): 514-518.

[12] M. Wiesinger, et al.  Rev. Sci. Instrum. 94.12 (2023).

[13] C. Will, et al. New J. Phys. 24.3 (2022): 033021.